
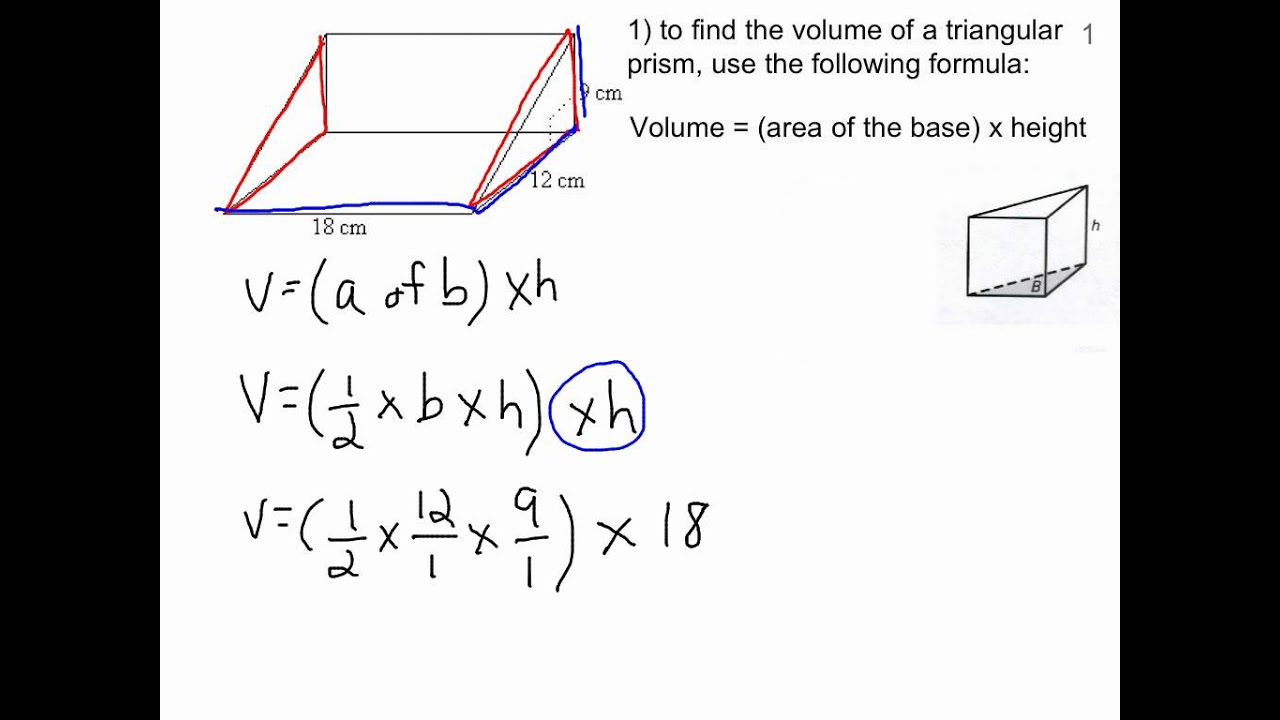
I redrew the figure from that page to make it clearer what the three equal-volume pyramids are: Here's how he divides the prism: A few propositions later, he extends the formula to cones. Given that, you can easily extend the formula to prisms with any shape base, since the base of any pyramid can be divided into triangles. The main part of the proof is to show that a triangular prism can be divided into three equal pyramids, so that the volume of one of the pyramids is one third of the volume of the prism that contains it. Uses no algebra, but more geometric reasoning. Next, I expanded on Euclid’s method: Now, Euclid's proof in: Dissecting a triangular prism into 3 triangular pyramids

The formula we ended up with is the correct one for a square pyramid, since the base area is \(B^2\). The more steps we used, the less stuff there is to cut off, so we can just ignore it. Technically, we have used calculus here, in the form of a limit but it’s a relatively natural kind of limit. (They represent the extra part of the steps that we have to cut off to get the pyramid.) Then the formula becomes: But if N is very large, 3/N and 1/N^2 are much smaller than 2, so we can ignore them. The formula for the volume of a pyramid is, in the most general form, $$V = \frac\right)$$ Now, if we use smaller and smaller steps (bigger and bigger N), our step pyramid will get closer and closer to the actual pyramid. How come the volume of a pyramid is equal to 1/3 the volume of a prism of the same base area and height? Can you explain it to me? The first question we’ll look at comes from Terence, in 1998: Volume of a Pyramid We’ll be seeing one method that comes very close to calculus (slicing and infinite series), and another that is fully geometrical (dissection, which we’ll do three different ways). Let’s do the same this time for a pyramid. Last week we looked at ways to derive the formulas for volume and surface area of a sphere, without using calculus.


 0 kommentar(er)
0 kommentar(er)
